Vectors and Kinematics – (Part 2 of 2)
You can find the first part of this series here: Vectors and Kinematics (Part 1 of 2).
Projectile Motion
Remember these acronyms: soh, cah, toa.
soh = sinº = opposite / hypotenuse
cah = cosº = adjacent / hypotenuse
toa = tanº = opposite / adjacent
- The opposite side is the one that is opposite the angle ‘x’
Example
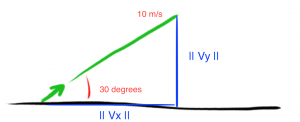
Consider a rocket is launched off at a 30º angle to the ground at 10 m/s.
Q. How far will it travel? (Horizontal displacement)
S denotes displacement
|| Vy || denotes magnitude of object in direction y
SOH, CAH, TOA
- sin(30) = || Vy || / 10 m/s
- 10 × sin(30) = || Vy ||
- 10 × ½ = || Vy ||
- 5 m/s = || Vy ||
← This is the vertical component
However, the vertical component goes up, as well as down.
Initial = Vi = 5 m/s
Final = Vf = -5 m/s
- Δvy = -5 m/s – 5m/s ⇒ -10 m/s
- Δvy = ay × Δt
- -10 m/s = -9.8 m/s2 × Δt
- Divide both sides by -9.8 m/s2
- ∴ 1.02 seconds = Δt
For the horizontal component:
- Cos(30) = || Vx || / 10 m/s
- 10 × Cos(30) = || Vx ||
- || Vx || = 5√3 m/s
- Sx = 5√3 m/
s× 1.02seconds - Sx = 8.83 meters
For the vertical component:
Vy = 5 m/s
Y axis meaning the up/down axis.
The positive value (+5 m/s) = the projectile is travelling up (y-axis); or to the right (x-axis). A negative value here would mean it was travelling downward (y-axis), or to the left (x-axis).
So, Vy = 5 m/s, meaning the object is travelling up @ 5 m/s
Q. How long does the rocket stay in the air?
- S = Vaverage × Δt
- S = (Vi + Vf)/2 × Δt
Vf = Vi + a × Δt
Deriving the formula:
- S = (Vi + Vi + a × Δt)/2 × Δt
- S = (Vi + a × Δt/2) × Δt
- S = Vi × Δt + (a +Δt2/2)
Now, at the start, S = 0
- 0 = 5 × Δt – (9.8(Δt2)/2)
- 0 = Δt × (5 – 4.9 × Δt) ← one of these underlined, must equal 0
- If we assume the first Δt = 0, then:
- 5 = 4.9 × Δt
- 5/4.9 = (
4.9× Δt)/4.9 - Δt = 1.02 seconds





You must be logged in to post a comment.